What Is Inequality?

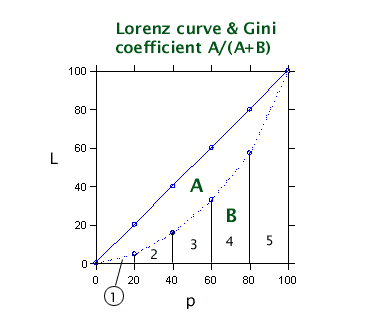
The Lorenz curve is the plot of the cumulative income share L against the cumulative population share p.

Can One Measure Inequality?

G = Area A/(Area A + Area B)

Thus the Gini coefficient in this example is 1786/5000 = 0.36 or 36%.
In the Lorenz curve the 45 degrees line represents a situation of perfect equality.  (Why?).
In general, the closer the Lorenz curve is to the line of perfect equality, the less the inequality and the smaller the Gini coefficient.

Countries differ in the inequality of their income distribution.

• Exhibit:  Comparison of Lorenz curves for 6 countries (Williamson 1991:12, Figure 1.2)

The following table shows the distribution of family wealth in the U.S. in 1983.  You may want to calculate the Gini coefficient as an exercise.
 

Inequality tends to be greater for wealth than for income.  (Why?).

• Exhibit:  Comparison of Lorenz curves for wealth and for income (U.S. 1983; from Kirbo)

• Exhibit: Fifteen wealthiest people in the world (Nolan and Lenski 2005)

Are There Other Ways to Measure Inequality?

1. Share of total income accruing to the top 20% of IRUs; this is 42.7 for U.S. family income distribution in 1983; share of the top 10%, or 5% are also used; the larger, the more unequal the distribution
2. Share of total income accruing to the bottom 40% of IRUs; this is 15.8 for U.S. family income distribution in 1983; the larger, the less unequal the distribution
3. Gap in constant $ between income of the 90th percentile and income of the 10th percentile; often used by economists; advantage is that knowledge of very top and very bottom incomes does not need to be accurate
4. Gini coefficient calculated form individual IRU data (as opposed to grouped data).  The formula is
G = (2/(μ*n²))Σ_{i=1 to n}i*x_i - (n+1)/n
where x_i is income, i is the rank of income x_i (i=1 for smallest income, i=n for largest), and μ is the mean income.
5. Theil coefficient (or "information theory" or "entropy" measure) calculated from individual IRU data.  The formula is
T = [(1/n)Σ_{i=1 to n}(x_i*log(x_i)) - μ*log(μ)]/μ
where log denotes the natural logarithm.
6. Varlog (variance of the logarithms of income) calculated from individual IRU data.  The formula is
V = (1/n)Σ_{i=1 to n}(z_i - z_.)²
where z_i = log(x_i) and z_. denotes the mean of the z_i
7. The coefficient of variation calculated from individual IRU data.  The formula is
C = s_X/μ
where s_X is the standard deviation of the x_i and μ is the mean of the x_i

• scale invariance: inequality is invariant to proportional increases or decreases in everyone's income (e.g., as may happen with inflation)
• principle of transfer: any transfer from an individual with a lower income to an individual with a higher income represents an increase in inequality, and vice versa

What is Dualism?

• inequality generated by the difference in average income between IRUs in the traditional /agricultural versus modern /urban sectors of a developing economy
• inequality generated by the difference in average income between black and white households in the U.S.

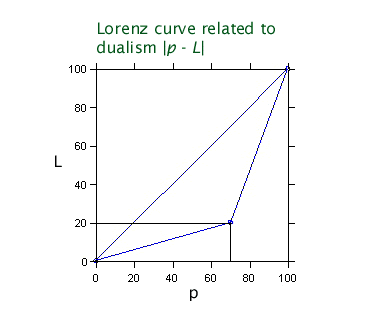
How is Dualism Measured?

D = |p - L|

where p is the percentage of IRUs in the poorest category and L is the percent income share of IRUs in the poorest category.  The symbol |  | denotes the absolute value.
The relationship of dualism to the Lorenz curve and the Gini coefficient is shown in the following exhibit.

Historically the concept of dualism was emphasized in a famous article by economist Simon Kuznets (1955) that initiated a stream of research on the relationship of income inequality with economic development.  Kuznets thought that dualism generated by income differences between traditional/agricultural and modern/urban sectors was a principal reason for the high level of income inequality in developing countries.  The following exhibit shows sector dualism figures for countries of the world around 1970.

• Exhibit: Income inequality (GIN) and sector dualism (SDU) for selected countries ca. 1970

The Big Picture: Inequality Trends and Sociocultural Evolution

Inequality has evolved in a systematic way during sociocultural evolution according to Lenski.

• Exhibit: Inequality and sociocultural evolution

Patterns of Inequality in the Modern World

1.  The Kuznets Curve

• Exhibit: The Kuznets curve: Income inequality by GDP/capita  (Alderson and Nielsen 1999, Figure 1)
• Exhibit: Inequality trajectories of industrial societies in the 20th century (Lindert & Williamson 1985, Figure 2 p. 345)
• Exhibit: Distribution of household incomes in industrial societies  (Nolan and Lenski 1999, Table 12.1 p. 291)

• the expansion of education (producing a linear decline in inequality with economic development)
• the shift from agriculture to the secondary (manufacturing) and tertiary (services) sectors (producing an inverted-U trend of inequality because of sector dualism)
• the demographic transition (also producing an inverted-U trend of inequality, with inequality highest at point of fastest population growth)

2.  The Great U-Turn in the U.S. and a Few Advanced Industrial Societies

• Exhibit: The Great U-Turn in the U.S.  (Nielsen and Alderson 1997, Figure 1)
• Exhibit: Income Inequality in the U.S. from 1960 to the late 1990s  (Scientific American June 1999 pp. 26-27)

• deindustrialization ( = decline in manufacturing employment) caused in part by globalization and international competition
• increasing reliance on technology causing increased demand (and higher returns) for education and cognitive skills
• increasing proportion of female-headed households

• Exhibit: The Changing Shape of the Nation's Income Distribution, 1947-98  (Jones and Weinberg 2000.)
• Exhibit: Other Census site location

• in 1993 the Census Bureau changed data collection procedures of the Current Population Survey to raise the maximum value of reported income.  This change resulted in higher inequality figures; inequality figures before and after 1993 are therefore not comparable.
• since 1993, inequality has not changed much (it has neither increased nor decreased substantally)

3. Current World Trends

Hans Rosling's Gapminder site provides graphic depictions of trends in income inequality in countries of the world.

Major trends:

• Inequality within developing countries is increasing
• Overall world inequality is decreasing because of economic development, especially of very large countries (China, India)

4.  Regional Inequality Patterns in North Carolina

• Exhibit: Race dualism in distribution of family income in North Carolina counties, 1980  (Nielsen and Warren 1998)
• Exhibit: Inequality in distribution of family income in North Carolina counties, 1980  (Nielsen and Warren 1998)
• Exhibit: Family income inequality by race dualism with DWLS nonparametric regression curve; North Carolina counties, 1980  (Nielsen and Warren 1998)

Discussion: Is Bill Gates Hazardous to Your Health?

• How can we distinguish an effect of inequality per se from the "absolute" effects of environmental characteristics, such as hunger or poverty?  E.g.,
• is the young man moved to break into the rich mansion because he is poor in absolute terms, or because he cannot stand the contrast between the luxurious lifestyle of the rich and his own modest circumstances?
• am I depressed because I have no money, or is my depression aggravated because I know Bill Gates has too much of it?
• It is possible that humans are so preoccupied with issues of inequality because evolution has given us a built-in "module" concerned with distributive justice, as evolutionary psychologists would argue (Trivers 1971, 1985: Chapter 15)
• Other important concepts are related to inequality but do not coincide with it, including
• poverty (measured as % below a given threshold of income)
• living standards (corresponding to some notion of average income)
• human rights (are individuals protected from torture, loss of life, loss of freedom at the hands of the powerful?)
• Is there a trade-off between inequality and economic development?

One study of the effect of income inequality on health outcomes at the county level finds a "modest independent effect [of income inequality] on the level of depressive symptoms, and on baseline and follow-up self-rated health, but no independent effect on biomedical morbidity or subsequent mortality"; these effects are substantially smaller than the effects of individual income.

• Fiscella and Franks (2000)

References

• Allison, Paul D.  1978.  "Measures of Inequality." American Sociological Review 43:865-80.
• Harrison, Bennett and Barry Bluestone.  1988.  The Great U-Turn: Corporate Restructuring and the Polarizing of America.  New York:  Basic Books.
• Kerbo, Harold R.  2000.  Social Stratification and Inequality.  New York: McGraw-Hill.
• Kuznets, Simon.  1955.  "Economic Growth and Income Inequality." American Economic Review 45:1-28
• Lecaillon, Jacques, Felix Paukert, Christian Morrisson, and Dimitri Germidis.  1984.  Income Distribution and Economic Development: An Analytical Survey.  Geneva, Switzerland: International Labour Office.
• Lindert, Peter H.  2000.  "Three Centuries of Inequality in Britain and America."  Pp. 167-216 in Handbook of Income Distribution, Volume 1, edited by Anthony B. Atkinson and François Bourguignon.  Amsterdam, Netherlands: Elsevier Science.
• Nielsen, François. 1994. "Income Inequality & Industrial Development: Dualism Revisited." American Sociological Review 59:654-677.
• Nielsen, François and Arthur S. Alderson. 1997. "The Kuznets Curve and the Great U-Turn: Income Inequality in U.S. Counties, 1970 to 1990." American Sociological Review 62:12-33.
• Nielsen, François and Arthur S. Alderson.  2001.  "Trends in Income Inequality in the United States."  Pp. 355-385 in Sourcebook on Labor Markets: Evolving Structures and Processes, edited by Ivar Berg and Arne L. Kalleberg.  New York: Plenum.
• Nielsen, François and Charles S. Warren. 1998. "Patterns of Income Inequality in North Carolina, 1980."  Sociological Analysis 1(3):87-112.
• Nolan, Patrick and Gerhard Lenski.  1999.  Human Societies: An Introduction to Macrosociology.  (8th edition.)  New

     York: McGraw-Hill.
• Nygård, Fredrik and Arne Sandström.  1981.  Measuring Income Inequality.  Stockholm, Sweden: Almquist and Wiskell.
• Trivers, Robert L.  1971.  "The Evolution of Reciprocal Altruism." Quarterly Review of Biology 46:35-57.
• Trivers, Robert L.  1985.  Social Evolution.  Menlo Park, CA: Benjamin / Cummings.